When you look at the moon through binoculars or a small telescope, the first thing you notice is that the lunar surface is divided into two distinct forms of terrain: large dark flat plains and bright mountainous highlands. Both of these are pockmarked by an enormous number of craters of all sizes.

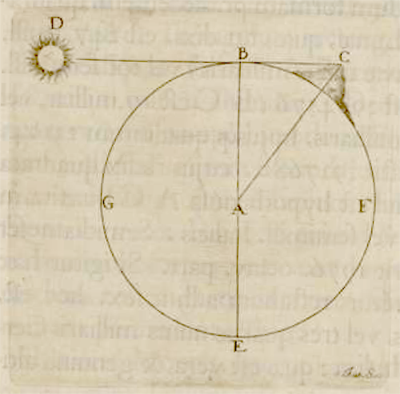
 The earliest method used by Galileo, Riccioli, and Hevelius in the mid-17th century, relied upon a game-changing realization:  the shifting pattern of light and dark at the Moon’s terminator is caused by sunlight interacting with peaks and valleys.  If a bright spot appears beyond the terminator, surrounded by blackness, it must mean that a mountain stands there, its height allowing the peak to catch the sun’s rays while everything around it is shadowed.  Hevelius took advantage of this situation to calculate the height of select mountains when the Moon was at quadrature – half illuminated as viewed from Earth. The illuminated hemisphere is bounded by the letters BGEA, but the hemisphere viewed by the observer is BGAF.  We see a disk half light and half dark, and a ray of light, DBC, just skims the edge of the Moon to hit the peak of a mountain at C.  This geometry is special because the ray of light is perpendicular to our line of sight.  That means that the distance between the terminator and the mountain (BC) can be directly compared to the diameter of the Moon because you’re seeing it straight on, while at any other angle this distance would be somewhat foreshortened. Once you have that ratio – the distance BC over the lunar diameter – you know the lengths of two sides of the right triangle, and all you have to do is calculate the hypotenuse, CA, and subtract the lunar radius to get the height of the mountain itself.